Electromagnetic radiation is characterized by a broad range of wavelengths and frequencies, each associated with a specific intensity (or amplitude) and quantity of energy. This interactive tutorial explores the relationship between frequency, wavelength, and energy, and enables the visitor to adjust the intensity of the radiation and to set the wave into motion.

The tutorial initializes with a visible light wave appearing in the window having a wavelength of 650 nanometers (red light) and amplitude of 61 candelas. Energies associated with waves in the tutorial appear beneath the window and are given in units of kJ/mole. To adjust the wavelength (and simultaneously, the frequency) of the wave, translate either the Wavelength or Frequency sliders to the left or right. As the sliders are relocated, the new values for wavelength and frequency appear above the sliders, the wave color changes to match the value for visible light associated with the wavelength, and the energy associated with the wave appears beneath the tutorial window. The amplitude of the wave can be adjusted with the Amplitude slider, and the resulting intensity values will appear above the slider, measured in units of candelas. In order to stop propagation of the wave, click on the Propagation Stop button located in the lower right-hand side of the tutorial window. The wave can be restarted by again clicking on the button, which changes into a Start button when the wave is halted.

An electromagnetic wave moves or propagates in a direction that is at right angles to the vibrations of both the electric and magnetic oscillating field vectors, carrying energy from its radiation source to undetermined final destination. The two fields are mutually perpendicular. By convention, and to simplify illustrations, the vectors representing the electric and magnetic oscillating fields of electromagnetic waves are often omitted, although they are understood to still exist.

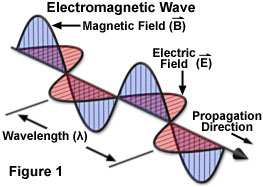
Whether transmitted to a radio from the broadcast station, heat radiating from the oven, furnace or fireplace, X-rays of teeth, or the visible and ultra-violet light emanating from the sun, the various forms of electromagnetic radiation all share fundamental wave-like properties. Every form of electromagnetic radiation, including visible light, oscillates in a periodic fashion with peaks and valleys, and displaying a characteristic amplitude, wavelength, and frequency that defines the direction, energy, and intensity of the radiation.

The standard unit for all electromagnetic radiation is the magnitude of the wavelength (in a vacuum), which is usually reported in terms of nanometers for the visible light portion of the spectrum. Each nanometer represents one-thousandth of a micrometer, and is measured by the distance between two successive peaks (see Figure 1). The corresponding frequency of the radiation wave, the number of sinusoidal cycles (oscillations or complete wavelengths) that pass a given point per second, is proportional to the reciprocal of the wavelength. Frequency is usually measured in Hertz (Hz) or cycles per second (cps). Thus, longer wavelengths correspond to lower frequency radiation and shorter wavelengths correspond to higher frequency radiation.

The different wavelengths and frequencies of various forms of electromagnetic radiation are fundamentally similar in that they all travel at the same speed--about 186,000 miles per second (approximately 300,000 kilometers per second), commonly known as the speed of light (and identified with the variable c). Electromagnetic radiation (including visible light) travels 149 million kilometers (93 million miles) from the sun to Earth in about 8 minutes. In contrast, an automobile speeding at 100 kilometers per hour (60 miles per hour) would require 177 years to make the same one-way trip. In only one second, light can circumnavigate the Earth seven times.

The wavelength of light, and all other forms of electromagnetic radiation, is related to the frequency by a relatively simple equation:

where c is the speed of light (measured in meters per second), n is the frequency of the light in hertz (Hz), and l is the wavelength of the light measured in meters. From this relationship one can conclude that the wavelength of light is inversely proportional to frequency. An increase in frequency produces a proportional decrease in the wavelength of light with a corresponding increase in the energy of the photons that compose the light. Upon entering a new medium (such as glass or water from air), the speed and wavelength of light is reduced, although the frequency remains unaltered.

---

BACK TO ELECTROMAGNETIC RADIATION

BACK TO LIGHT AND COLOR

Questions or comments? Send us an email.

© 1998-2022 by Michael W. Davidson and The Florida State University. All Rights Reserved. No images, graphics, scripts, or applets may be reproduced or used in any manner without permission from the copyright holders. Use of this website means you agree to all of the Legal Terms and Conditions set forth by the owners.

This website is maintained by our
Graphics & Web Programming Team
in collaboration with Optical Microscopy at the
National High Magnetic Field Laboratory.

Last modification: Sunday, Feb 28, 2016 at 10:36 AM

Access Count Since October 4, 2002: 470370

For more information on microscope manufacturers,
use the buttons below to navigate to their websites: